Earliest Uses of Symbols of Set Theory and Logic

Most of the basic symbols of logic and set theory in use today were introduced between 1880 and 1920. The main contributors were Ernst Schröder (1841-1902), Giuseppe Peano (1858-1932), Alfred North Whitehead (1861-1947) and Bertrand Russell (1872-1970). Peano had a strong influence on Whitehead and Russell and their joint work, Principia Mathematica (1910-1913), was itself very influential. Today Gottlob Frege (1848-1925) is the most admired logician of that age but his notation was not taken up. Most of the symbols described here are treated in the chapter on mathematical logic in Cajori's A History of Mathematical Notations, vol. 2 (1929). The ideas of the period are covered in I. Grattan-Guinness (2000) The Search for Mathematical Roots, 1870-1940: Logics, Set Theories and the Foundations of Mathematics from Cantor through Russell to Gödel. Many of the classic works are available in English in Jan van Heijenoort (1967) From Frege to Gödel: A Source Book in Mathematical Logic, 1879-1931.

For set theory and logic entries on the Words pages, see here for a list.

Intersection and union. The symbols ∩ and ∪ were first used by Hermann Grassmann in Die Ausdehnungslehre von 1844, §2+§5. There Grassmann used the two symbols still as general operation symbols, not specialized for intersection and union. [Wilfried Neumaier]. From there Giuseppe Peano (1858-1932) took both symbols and used them for intersection and union in 1888 in Calcolo geometrico secondo l'Ausdehnungslehre di H. Grassmann (Cajori vol. 2, page 298); the logical part of this work with this symbols is ed. in: Peano, opere scelte, 2, Rom 1958, p. 3-19.

Peano also created the large symbols for general intersection and union of more than two classes in 1908. These symbols can be seen in the Wikipedia article on Union (set theory). The source is: G. Peano: Formulario mathematico, tomo V, Torino 1908, Facsimile-Reprint, Rom 1960, p. 82. [This information was provided by Wilfried Neumaier, who reports that Cajori vol. 2 does not have the correct earliest use for these symbols.]

Existence (existential quantifier). Peano used in volume II, number 1, of his Formulaire de mathematiqués, which was published in 1897 (Cajori vol. 2, page 300).

Kevin C. Klement writes, "While Peano had the backwards E for a predicate of classes, Russell was the first to use the backwards E as a variable binding operator, and there are the wonderful manuscripts printed in CPBR vol 4 in which Russell's makes large dots out of Peano's backwards epsilons to change over from the Peano-notation for existence to a more Fregean one."

Membership. Peano used for membership in “Arithmetices prinicipia nova methodo exposita,” 1889; ed. in: Peano, opere scelte, 2, Rom 1958, p. 27. [This citation was contributed by Wilfried Neumaier.] The website at the University of St. Andrews states that Peano introduced the symbol in 1889 and that it comes from the first letter of the Greek word meaning "is."

Peano also used this symbol in the introduction to volume I of his Formulaire de mathematiqués, which was published in Turin in 1895, although the introduction itself is dated 1894 (Cajori vol. 2, page 300).

Peano's symbol for membership was an ordinary epsilon ; the stylized epsilon now used was adopted by Bertrand Russell in Principles of Mathematics in 1903 (Julio González Cabillón).

Kevin C. Klement writes, "I think Russell always intended to use epsilon, but the way both POM and PM are typeset, it does come off looking more like the modern membership sign. Certainly Russell's manuscripts after 1903 continue to use epsilon. Is typographical accident the root of the modern sign?"

Such that. According to Julio González Cabillón, Peano introduced the backwards lower-case epsilon for "such that" in "Formulaire de Mathematiques vol. II, #2" (p. iv, 1898).

Peano introduced the backwards lower-case epsilon for "such that" in his 1889 "Principles of arithmetic, presented by a new method," according van Heijenoort's From Frege to Gödel: A Source Book in Mathematical Logic, 1879--1931 [Judy Green].

For all. According to M. J. Cresswell and Irving H. Anellis, 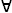originated in Gerhard Gentzen, "Untersuchungen ueber das logische Schliessen," Math. Z., 39, (1935), p, 178. In footnote 4 on that page, Gentzen explains how he came to use the sign. It is the "All-Zeichen," an analogy with for the existential quantifier which Gentzen says that he borrowed from Russell.

Cajori, however, shows that Peano used before Russell and Whitehead (whose backwards E had serifs, unlike Peano's).

Russell used the notation (x) for "for all x". See his "Mathematical Logic as Based on the Theory of Types," American Journal of Mathematics, 30, (1908), 222-262. [Denis Roegel].

Braces enclosing the elements of a set. Georg Cantor used the set brackets {a}, {a,b},... in 1878 in “Ein Beitrag zur Mannifaltigkeitslehre” in Crelles Journal für Mathematik, 84 (1878), p. 242-258. [This citation was contributed by Wilfried Neumaier.]

Cantor later used this symbolism as follows:

Unter einer 'Menge' verstehen wir jede Zusammenfassung M von bestimmten wohlunterschiedenen Objecten m unsrer Anschauung oder unseres Denkens (welche die 'Elemente' von M genannt werden) zu einem Ganzen.

In Zeichen druecken wir dies so aus:

M = {m}.

M stands for the German term "Menge." Cantor may have used this notation earlier in his correspondence with the mathematicians of his day. (This entry was contributed by Julio González Cabillón.)

p is used for propositions in 1897 in Peano's "Studii di logica matematica," according to Kevin C. Klement.

p, q, and r were used as propositional letters by Bertrand Russell in 1903 in The Principles of Mathematics (OED).

Negation. The tilde ~ for negation was used by Peano in 1897. See Peano, “Studii di logica matematica,” ed. in: Peano, opere scelte, 2, Rom 1958, p. 212. [Kevin C. Klement]

~p for "the negation of p" appears in 1908 in the article "Mathematical logic as based on the theory of types" by Bertrand Russell [Denis Roegel].

The symbolism was also used in 1910 by Alfred North Whitehead and Bertrand Russell in the first volume of Principia mathematica (Cajori vol. 2, page 307).

The main symbol for negation wich is used today is ¬. It was first used in 1930 by Arend Heyting in “Die formalen Regeln der intuitionistischen Logik,” Sitzungsberichte der preußischen Akademie der Wissenschaften, phys.-math. Klasse, 1930, p. 42-65. The ¬ appears on p. 43. [Wilfried Neumaier]

Disjunction. ∨ for disjunction is found in Russell's manuscripts from 1902-1903 and in 1906 in Russell's paper "The Theory of Implication," in American Journal of Mathematics vol. 28, pp. 159-202, according to Kevin C. Klement.

p \/ q for "p or q" appears in 1908 in the article (1908) "Mathematical Logic as Based on the Theory of Types," American Journal of Mathematics, 30, 222-262. by Bertrand Russell [Denis Roegel].

The symbolism was also used in 1910 by Alfred North Whitehead and Bertrand Russell in the first volume of Principia mathematica. (These authors used p.q for "p and q.") (Cajori vol. 2, page 307)

Conjunction. The symbol ∧ for logical conjunction "and" was first used in 1930 by Arend Heyting in the same source as shown for the negation symbol above. [Wilfried Neumaier]

Implication. The arrow symbol → for the logical implication was first used in 1922 by David Hilbert in: Hilbert: Neubegründung der Mathematik, 1922, in: Abhandlungen aus dem Mathematischen Seminar der Hamburger Universität, Band I (1922), 157-177. The symbol is found on p. 166. [Wilfried Neumaier]

The arrow with double lines ⇒ was first used 1954 by Nicholas Bourbaki, in: Bourbaki: Theorie des ensembles, 3. edition, Paris, 1954. The symbol appears on p. 14. [Wilfried Neumaier]

Equivalence. The double arrow symbol ↔ for the logical equivalence was apparently first used in 1936 by Wilhelm Ackermann in: Ackermann, W.: Die Widerspruchsfreiheit der allgemeinen Mengenlehre, in: Mathematische Annalen 114 (1937), 305-315. The symbol appears on p. 306. [Wilfried Neumaier]

The double arrow with double line ⇔ was first used 1954 by Nicholas Bourbaki, in: Bourbaki: Theorie des ensembles, 3. edition, Paris, 1954. The symbol appears on p. 32. [Wilfried Neumaier]

The null set symbol (Ř) first appeared in N. Bourbaki Éléments de mathématique Fasc.1: Les structures fondamentales de l'analyse; Liv.1: Theorie de ensembles. (Fascicule de resultants) (1939): "certaines propriétés... ne sont vraies pour aucun élément de E... la partie qu’elles définissent est appelée la partie vide de E, et designée par la notation  Ř." (p. 4.)

André Weil (1906-1998) says in his autobiography that he was responsible for the symbol:

Wisely, we had decided to publish an installment establishing the system of notation for set theory, rather than wait for the detailed treatment that was to follow: it was high time to fix these notations once and for all, and indeed the ones we proposed, which introduced a number of modifications to the notations previously in use, met with general approval. Much later, my own part in these discussions earned me the respect of my daughter Nicolette, when she learned the symbol Ø for the empty set at school and I told her that I had been personally responsible for its adoption. The symbol came from the Norwegian alphabet, with which I alone among the Bourbaki group was familiar.

This letter is used in the Norwegian, Danish and Faroese alphabets.

The "therefore" symbol ()was first published in 1659 in the original German edition of Teusche Algebra by Johann Rahn (1622-1676) (Cajori vol. 1, page 212, and vol 2., page 282).

The "because" symbol (). Cajori writes, "We have not been able to find the use of for 'because' in the eighteenth century. This usage seems to have been introduced in Great Britain and the United States in the nineteenth century. It is found in the Gentleman's Mathematical Companion (1805). It did not meet with as wide acceptance in Great Britain and America as did for 'therefore'" (pages 282-283).

The halmos (a box indicating the end of a proof). On the last page of his autobiography, Paul R. Halmos writes:

My most nearly immortal contributions are an abbreviation and a typographical symbol. I invented "iff", for "if and only if" -- but I could never believe that I was really its first inventor. I am quite prepared to beieve that it existed before me, but I don't know that it did, and my invention (re-invention?) of it is what spread it thorugh the mathematical world. The symbol is definitely not my invention -- it appeared in popular magazines (not mathematical ones) before I adopted it, but, once again, I seem to have introduced it into mathematics. It is the symbol that sometimes looks like [an empty square], and is used to indicate an end, usually the end of a proof. It is most frequently called the "tombstone", but at least one generous author referred to it as the "halmos".

The aleph null symbol was conceived by Georg Cantor (1845-1918) around 1893, and became widely known after "Beiträge zur Begründung der transfiniten Mengenlehre"  [Contributions to the Foundation of Transfinite Set Theory] saw the light in Mathematische Annalen, Band XLVI [vol. 46], B. G. Teubner, Leipzig, 1895.

On page 492 of this prestigious journal we find the paragraph Die kleinste transfinite Cardinalzahl Alef-null [The minimum transfinite cardinal number Aleph null], and the following:

...wir nennen die ihr zukommende Cardinalzahl, in Zeichen, ... [We call the cardinal number related to that (set); in symbol, ]

In a letter dated April 30, 1895, Cantor wrote, "it seemed to me that for this purpose, other alphabets were [already] over-used" (translation by Martin Davis).

In Georg Cantor, Dauben (page 179) says that Cantor did not want to use Roman or Greek alphabets, because they were already widely used, and "His new numbers deserved something unique. ... Not wishing to invent a new symbol himself, he chose the aleph, the first letter of the Hebrew alphabet...the aleph could be taken to represent new beginnings...." Avinoam Mann points out that aleph is also the first letter of the Hebrew word "Einsof," which means infinity and that the Kabbalists use "einsof" for the Godhead.

Although his father was a Lutheran and his mother was a Roman Catholic, Cantor had at least some Jewish ancestry.

(Julio González Cabillón. contributed to this entry.)

Set inclusion. According to Cajori (vol. 2, page 294), the symbols  for "is included in" (untergeordnet) and for "includes" (übergeordnet) were introduced  by Schröder Vorlesungen über die Algebra der Logik vol. 1 (1890). Previously the symbols < and > had been used.

According to a web page by Paul Taylor, "Joseph Gergonne introduced C for contient and É for its converse in 1817, and these symbols were used by Peano and by Russell and Whitehead. (In fact Russell and Whitehead also used Ě for containment in our sense.)"

According W. V. Quine, Methods of Logic, 4th ed., Harvard University Press, 1982, page 132: “The inclusion signs and , now current in set theory, are derived from Gergonne's use in 1816 of 'C' for containment.” In the bibliography he lists the following: Gergonne, J. D. “Essai de dialectique rationelle.” Annales de mathématiques pure et appliquées, vol. 7 (1816-17), pp. 189-228. [Susanna Epp]

Equal by definition. =_Def is found in C. Burali-Forti Logicamatematica (1894) (Grattan-Guinness (2000) p. 216).